A team of engineers were required to measure the height of a flag pole. They only had a measuring tape, and were getting quite frustrated trying to keep the tape along the pole. It kept falling down, etc. A mathematician comes along, finds out their problem, and proceeds to remove the pole from the ground and measure it easily. When he leaves, one engineer says to the other: "Just like a mathematician! We need to know the height, and he gives us the length!"

What is "pi"?
Mathematician: Pi is the number expressing the relationship between the circumference of a circle and its diameter.
Physicist: Pi is 3.1415927 plus or minus 0.00000005
Engineer: Pi is about 3.

"A mathematician is a device for turning coffee into theorems" -- P. Erdos

Here's a limerick:

Which, of course, translates to:

Integral t-squared dt
from 1 to the cube root of 3
times the cosine
of three pi over 9
equals log of the cube root of 'e'.

And it's correct, too.

Another one:

In arctic and tropical climes,
the integers, addition, and times,
taken (mod p) will yield
a full finite field,
as p ranges over the primes.

The ark lands after The Flood. Noah lets all the animals out. Says, "Go and multiply." Several months pass. Noah decides to check up on the animals. All are doing fine except a pair of snakes. "What's the problem?" says Noah. "Cut down some trees and let us live there", say the snakes. Noah follows their advice. Several more weeks pass. Noah checks on the snakes again. Lots of little snakes, everybody is happy. Noah asks, "Want to tell me how the trees helped?" "Certainly", say the snakes. "We're adders, and we need logs to multiply."

Three men are in a hot-air balloon. Soon, they find themselves lost in a canyon somewhere. One of the three men says, "I've got an idea. We can call for help in this canyon and the echo will carry our voices far."
So he leans over the basket and yells out, "Helllloooooo! Where are we?" (They hear the echo several times).
15 minutes later, they hear this echoing voice: "Helllloooooo! You're lost!!"
One of the men says, "That must have been a mathematician." Puzzled, one of the other men asks, "Why do you say that?" The reply: "For three reasons.
(1) he took a long time to answer,
(2) he was absolutely correct, and
(3) his answer was absolutely useless."

1. What's the contour integral around Western Europe?

Answer: Zero, because all the Poles are in Eastern Europe!

Do you believe in one God?
Answer: Yes, up to isomorphism!

It's a city that can be guarded by finitely many near-sighted policemen!

Q: What's purple and commutes?
A: An abelian grape.

Q: What's yellow, and equivalent to the Axiom of Choice?
A: Zorn's Lemon.

The great logician Betrand Russell (or was it A.N. Whitehead?) once claimed that he could prove anything if given that 1+1=1. So one day, some smarty-pants asked him, "Ok. Prove that you're the Pope." He thought for a while and proclaimed, "I am one. The Pope is one. Therefore, the Pope and I are one."

Lemma: All horses are the same color.

Proof (by induction):

Case n=1: In a set with only one horse, it is obvious that all horses in that set are the same color.
Case n=k: Suppose you have a set of k+1 horses. Pull one of these horses out of the set, so that you have k horses. Suppose that all of these horses are the same color. Now put back the horse that you took out, and pull out a different one. Suppose that all of the k horses now in the set are the same color. Then the set of k+1 horses are all the same color. We have k true
=> k+1 true; therefore all horses are the same color.

Proof (by intimidation):

Everyone would agree that all horses have an even number of legs. It is also well-known that horses have forelegs in front and two legs in back. 4 + 2 = 6 legs, which is certainly an odd number of legs for a horse to have! Now the only number that is both even and odd is infinity; therefore all horses have an infinite number of legs.

However, suppose that there is a horse somewhere that does not have an infinite number of legs. Well, that would be a horse of a different color; and by the Lemma, it doesn't exist.


QED

Several students were asked the following problem:

Prove that all odd integers are prime.

Well, the first student to try to do this was a math student. Hey says "hmmm... Well, 1 is prime, 3 is prime, 5 is prime, and by induction, we have that all the odd integers are prime."

Of course, there are some jeers from some of his friends. The physics student then said, "I'm not sure of the validity of your proof, but I think I'll try to prove it by experiment." He continues, "Well, 1 is prime, 3 is prime, 5 is prime, 7 is prime, 9 is ... uh, 9 is an experimental error, 11 is prime, 13 is prime... Well, it seems that you're right."

The third student to try it was the engineering student, who responded, "Well, actually, I'm not sure of your answer either. Let's see... 1 is prime, 3 is prime, 5 is prime, 7 is prime, 9 is ..., 9 is ..., well if you approximate, 9 is prime, 11 is prime, 13 is prime... Well, it does seem right."

Not to be outdone, the computer science student comes along and says "Well, you two sort've got the right idea, but you'd end up taking too long doing it. I've just whipped up a program to REALLY go and prove it..." He goes over to his terminal and runs his program. Reading the output on the screen he says, "1 is prime, 1 is prime, 1 is prime, 1 is prime...."

A biologist, a statistician and a mathematician are on a photo-safari in africa. They drive out on the savannah in their jeep, stop and scout the horizon with their binoculars.

The biologist : "Look! There's a herd of zebras! And there, in the middle : A white zebra! It's fantastic ! There are white zebra's ! We'll be famous !"

The statistician : "It's not significant. We only know there's one white zebra."

The mathematician : "Actually, we only know there exists a zebra, which is white on one side."

1 + 1 = 3, for large values of 1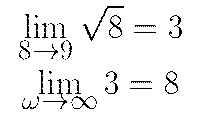

Theorem : All positive integers are equal.

Proof :

Sufficient to show that for any two positive integers, A and B, A = B. Further, it is sufficient to show that for all N > 0, if A and B (positive integers) satisfy (MAX(A, B) = N) then A = B.

Proceed by induction.

If N = 1, then A and B, being positive integers, must both be 1. So A = B.

Assume that the theorem is true for some value k. Take A and B with MAX(A, B) = k+1. Then MAX((A-1), (B-1)) = k. And hence (A-1) = (B-1). Consequently, A = B.

Theorem:
All positive integers are interesting.
Proof:
Assume the contrary. Then there is a lowest non-interesting positive integer. But, hey, that's pretty interesting! A contradiction.
QED

The shortest math joke ever: let epsilon < 0 (*)

Two functions meet in a narrow street. (*)

F1: Clear the way!
F2: No, I won't.
F1: Move over, or I will differentiate you!
F2: Ok, try it, I am the exp-function!

Click here to return to the main page